Peak Dispersion in a Chromatographic Column

The first comprehensive approach to dispersion in chromatographic columns was taken by Van Deemter (8) who developed the dispersion equation for a packed GC column. Van Deemter’s development did not take into account the compressibility of the mobile phase which was dealt with later by Katz, Ogan and Scott (9). A simple form of this theory will be given that does not accommodate the compressibility of the mobile phase but a more detailed and comprehensive treatment is given in Dispersion in Chromatography Columns and Extra Column Dispersion.

Van Deemter et al. assumed that there were four band spreading processes responsible for peak dispersion, namely, multi-path dispersion, longitudinal diffusion, resistance to mass transfer in the mobile phase and resistance to mass transfer in the stationary phase. Van Deemter derived an expression for the variance contribution of each process to the overall variance per unit length of the column. Furthermore, as the individual dispersion processes can be assumed to be random and non-interacting, the total variance per unit length of the column can be obtained from a sum of the individual variance contributions.

The Multi-Path Effect

The multi-path effect is diagramatically depicted in figure 20.

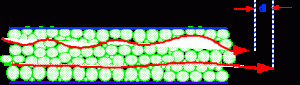
Figure 20 Multipath Dispersion

When flowing through a packed column, the individual solute molecules will describe a tortuous path through the interstices between the particles. As a result, some will randomly travel shorter routes than the average and some longer. It follows, that those molecules taking shorter paths will move ahead of the mean and those that take the longer paths will lag behind the mean. This will result in a differential distance traveled (dl) as shown in figure 3. This differential flow of the solute molecules results in band dispersion.